In chapter 6, we leave trigonometric functions (I can't be the only one glad to do so, right?) and move on to exponential functions. The simplest exponential function is in the form of y = ax, a > 0, a ≠ 1. There is a restrictions because if a≤0, you may not get a real number when you substitute it into the equation.
Let's study the function y = 2x and its graph. The base is 2 while the power x is the variable. After evaluating the function and determining the values, a graph of the exponential function can be drawn as shown.
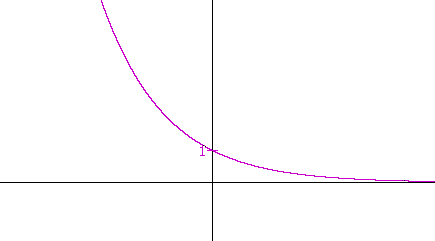
Here are some properties of the exponential function when the base is greater than 1.
The graph is decreasing when the base of an exponential functions is between 0 and 1.
- The domain is all real numbers
- The range is all real numbers and y>0.
- has y-intercept of 1 as the graph passes through the point (0,1)
- has a horizontal asymptote at y=0
- The graph is increasing as a > 1
The graph is decreasing when the base of an exponential functions is between 0 and 1.
