Logarithm study has a few formulas that are important and key to solving math questions. By remembering them, you will be in line to solve logarithmic problems and, maybe, fast too. There are a few logarithms laws that are formed by combining two logs of the same base.
- Product rule : log(ab) = log(a) + log(b)
- Quotient rule : log(a/b) = log(a) - log(b)
- Power rule : log(xn) = nlog(x) Ex: log39² = 2log39
If the logarithm to the base a is known, then the logarithm to the base b can be obtained by the base change relationship:
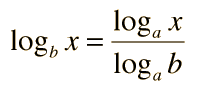
No comments:
Post a Comment